- Since we are in characteristic zero, everything is separable
so we may use the theorem of the
primitive element. This tells us that there exists
a
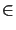 K
such that
K = F(a). If p is the minimal polynomial of
a over F, then
K
K
such that
K = F(a). If p is the minimal polynomial of
a over F, then
K 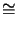 F[X]/(p).
F[X]/(p).
- Since p is the minimal polynomial, it is monic and irreducible
in F[X]. Also we are in characteristic zero, so p is separable.
This means that when we write
p = p1...pn in K[X] where the
pi are monic irreducible polynomials, the pi are distinct.
Now write
c = f[X] + (p) where
f
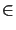 K[X]. Since c2 = 0, we
see that
f2
K[X]. Since c2 = 0, we
see that
f2 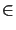 (p). Thus
f2 = p1...pnq for some
q
(p). Thus
f2 = p1...pnq for some
q 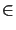 K[X]. By uniqueness of factorization, we have pi divides f for
all i and we deduce that f
K[X]. By uniqueness of factorization, we have pi divides f for
all i and we deduce that f 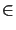 (p). Therefore c = 0 as
required.
(p). Therefore c = 0 as
required.
- Suppose the action of M
2(
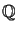 ) has a finite orbit with at
least two elements. Using
the formula that the number of elements in an orbit is the index of
the stablizer of any element in that orbit, we see that M
2(
) has a finite orbit with at
least two elements. Using
the formula that the number of elements in an orbit is the index of
the stablizer of any element in that orbit, we see that M
2(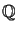 ) has a nontrivial subgroup H of finite index. Then qM
2(
) has a nontrivial subgroup H of finite index. Then qM
2(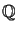 )
) 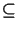 H for some positive integer q.
However if
a
H for some positive integer q.
However if
a 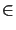 M
2(
M
2(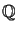 ), then
a = q(a/q), which shows that qM
2(
), then
a = q(a/q), which shows that qM
2(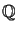 ) = M
2(
) = M
2(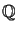 ). We
conclude that M
2(
). We
conclude that M
2(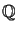 ) = H and the result follows.
) = H and the result follows.
- To check that we have an action, we must show that
(gh)*l = g*(h*l) for all g, h
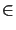 GL
2(
GL
2(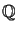 ). This is true
because
). This is true
because
(g*h)(l) = det(gh)l/| det(gh)| = det(g)det(h)l/(| det(g)|| det(h)|) = det(g)(h*l)/| det(g)| = g*(h*l).
Finally we see that all orbits have the form {±l}, and so in particular there are finite orbits which are not singletons.
- First H has an identity, namely the zero homomorphism defined
by 0(a) = 0 for all a
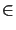 A. If
f, g
A. If
f, g 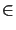 H, then
H, then
(f + g)(a + b) = f (a + b) + g(a + b) = f (a) + f (b) + g(a) + g(b) = f (a) + g(a) + f (b) + g(b) = (f + g)(a) + (f + g)(b)
which shows that f + g is a homomorphism, and so f + g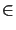 H.
Also if
f, g, h
H.
Also if
f, g, h 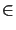 H, then
H, then
((f + g) + h)(a) = (f + g)(a) + h(a) = f (a) + g(a) + h(a) = f (a) + (g + h)(a) = (f + (g + h))(a),
so (f + g) + h = f + (g + h) which establishes the associative law. Finally for f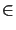 H, the inverse of f is - f, where
(- f )(a) = - f (a). Since
(- f )(a + b) = - f (a + b) = - f (a) - f (b) = (- f )(a) + (- f )(b), we see that - f
H, the inverse of f is - f, where
(- f )(a) = - f (a). Since
(- f )(a + b) = - f (a + b) = - f (a) - f (b) = (- f )(a) + (- f )(b), we see that - f 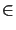 H, and we have established that H is a group.
H, and we have established that H is a group.
- We first show that H is torsion free. If f
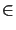 H has order
n
H has order
n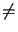 1, then
f (a)
1, then
f (a)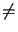 0 for some a
0 for some a 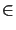 A. But then
(nf )(a) = n(fa)
A. But then
(nf )(a) = n(fa)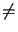 0, a contradiction. Therefore
the subgroup generated by
f1,..., fm is a finitely generated torsion free abelian group, so by
the fundamental structure theorem for finitely generated abelian
groups it is free.
0, a contradiction. Therefore
the subgroup generated by
f1,..., fm is a finitely generated torsion free abelian group, so by
the fundamental structure theorem for finitely generated abelian
groups it is free.
- A 2 by 2 matrix with entries in
 /p
/p will
be invertible if and only if its columns are linearly independent over
will
be invertible if and only if its columns are linearly independent over
 /p
/p . So there are p2 - 1 choices for the
first column (we cannot choose (0, 0) for the first column) and p2 - p choices for the second column (we cannot choose the vector in the
first column). It follows that
| G| = (p2 - 1)(p2 - p).
. So there are p2 - 1 choices for the
first column (we cannot choose (0, 0) for the first column) and p2 - p choices for the second column (we cannot choose the vector in the
first column). It follows that
| G| = (p2 - 1)(p2 - p).
- Note that H is a Sylow p-subgroup of G, so the number of conjugates of H is congruent to 1 modulo p. Therefore 1 is congruent to 8 modulo p, which can only happen if p = 7. In the case p = 7, we have by Sylow's theorem that the number of conjugates of H in G is congruent to 1 modulo 7, which is of course congruent to 8 modulo 7. Thus the answer is p = 7.