Algebra Prelim, Fall 1986

1. 1. Let G be a finite abelian (multiplicative) group. Prove that if G is not cyclic, then there exists a positive integer n such that n< |G| and gⁿ = e for all .
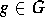   2. Prove that the multiplicative group of a finite field is cyclic.
2. Let G be a group of order , and let H be a subgroup of order .
   1. Prove that H is abelian.
   2. Prove that H is normal in G.
   3. Prove that G is abelian.
3. Let R be an integral domain. For an element , prove the equivalence of the following two statements.
   1. There exists an infinite chain 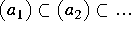of principal ideals of R with a=a₁.
   2. There exists an infinite set 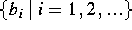of nonunits of R such that b₁ b₂ ... b_n divides a for each positive integer n.
4. Let R be a commutative ring with identity and let M be a maximal ideal of R.
   1. Prove that .
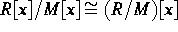   2. Conclude that M[x] is a prime ideal but not a maximal ideal in R[x]. Indeed argue that there are infinitely many prime ideals of R[x] which contain M[x].
5. Let be fields such that K is a splitting field over k. If , prove that .
6. Let K/k be a finite extension and let with . If , prove that n | [K:k].
7. Let f(x) = xⁿ-1 and let K be a splitting field for f(x) over . Prove that the Galois group is abelian.
8. Let M be a module. A submodule S of M is small if whenever S+N= M for any submodule N of M, then N=M. Suppose S is small in M and there exists an epimorphism where P is projective. Prove that there exists an epimorphism .

Return to

• Algebra Prelims Index
• Peter Linnell's Homepage

 
• About this document ...