- Define what is meant by a prime ideal in a commutative ring.
- Prove that a nonzero prime ideal in a principal ideal domain is
always a maximal ideal.
- Prove that I is an ideal of R.
- Show that either
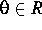 or there exists a maximal ideal
or there exists a maximal ideal
 of R such that
of R such that 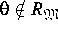 .
.
- Conclude that
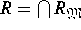 , where the
intersection is taken over all the maximal ideals of R.
, where the
intersection is taken over all the maximal ideals of R.
- H and K are nilpotent groups,
- there are homomorphisms
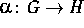 and
and  , and
, and
-
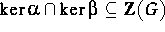 , the center of
G.
, the center of
G.