Algebra Preliminary Exam, Fall 1982

Do all problems

1. Prove that a group of order has a normal subgroup of order 15.
2. 1. For a positive integer n, show that every ideal in 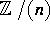is principal.
   2. Explain how one determines the number of ideals of 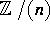in terms of n.
3. 1. Calculate the Galois group of (x²-2)(x²+3) over .
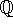   2. Explicitly state the correspondence between the subfields of the splitting field K of (x²-2)(x²+3) over 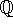and the subgroups of .
4. Let V and W be vector spaces over the field k and let W^* be the space of linear functions from W to k. Prove that the map defined by is
   1. Well defined.
   2. Linear.
5. Let K be a field of characteristic . Suppose f(x) = p(x)/q(x) is a ratio of polynomials in K[x]. Prove that if f(x) = f(-x), then there are polynomials p₀(x²), q₀(x²) such that f(x) = p₀(x²)/q₀(x²). (HINT: look for a field automorphism of K(x) that fixes f(x).)
6. Let G be a solvable group. Prove that if is normal in G and contains no other non trivial subgroups which are normal in G, then N is abelian.
7. Let A,B,C,D be finite abelian groups such that and . Prove that .

Return to

• Algebra Prelims Index
• Peter Linnell's Homepage

 
• About this document ...