Algebra Prelim Solutions, Fall 2003

1. Let G be a simple group of order 2256 = 16*3*47. The number of Sylow 47-subgroups is congruent to 1 mod 47 and divides 48. Since G is simple, the number of Sylow 47-subgroups is not 1 (otherwise G would have a normal Sylow 47-subgroup), consequently G has 48 Sylow 47-subgroups. Next the number of Sylow 3-subgroups is congruent to 1 mod 3 and divides 16*47. This number cannot be 1 because G is simple. Nor can it be 4, because then G would be isomorphic to a subgroup of A₄ which only has order 12. We conclude that G has at least 16 Sylow 3-subgroups. Finally G has at least two Sylow 2-subgroups. Note that two distinct Sylow 47-subgroups P, Q intersect trivially, because | P /\ Q| divides 47 and is not 47. Similarly two distinct Sylow 3-subgroups intersect trivially. On the other hand we cannot assert that two distinct Sylow 2-subgroups intersect trivially.

   We now count elements. The number of elements of order 47 is 46*48; the number of elements of order 3 is at least 2*16, and the number of elements of order a power of two is at least 17. We now have at least 17 + 2*16 + 46*48 = 2257 elements in G, which is too many. This proves that there is no simple group of order 2256.

2. (i)

   Every element of G can be written in the form x^a₁y^b₁...x^a_ny^b_n, where a_i, b_i $\in$ Z. Using x⁷ = y³ = 1, we may assume that a_i, b_i> 0 for all i. Now whenever there is a subword yx, we can replace it with xy², because yxy^-1 = x², so we may push all the x's to the left and all the y's to the right. This proves the result.

   (ii)

   Using (i) and x⁷ = y³ = 1, we may write every element of G in the form xⁱy^j where 0 < i < 6 and 0 < j < 2. It follows that | G| < 7*3 = 21.

   (iii)

   This is because q respects the relations. Specifically, (1 3 4 5 6 7)⁷ = 1, ((2 3 5)(4 7 6))³ = 1,

   ((2 3 5)(4 7 6))(1 2 3 4 5 6 7)((2 3 5)(4 7 6))^-1 = (1 3 5 7 2 4 6).

   (iv)

   We see from (iii) that imq has elements of order 7 and 3. Therefore 21 divides | imq| and hence 21 divides | G|. Since | G| < 21 from (ii), we deduce that | G| = 21 as required.

3. We may assume that a, b =/=  0. Let d be the greatest common divisor of a, b. We claim that a/d, b/d are coprime. If r divides a/d, b/d, then rd divides a, b and hence rd divides d, say srd = d for some s $\in$ R. Therefore sr = 1, which means that r is a unit and the claim is established. By hypothesis (a/d )R + (b/d )R = xR for some x $\in$ R. Then aR + bR = xdR and the result follows.

4. Note that M @ Z/2Z as R-modules, so we need to prove Z/2Z $\otimes_{R}^{}$ Z/2Z @ Z/2Z. Define q : Z/2Z $\otimes_{R}^{}$ Z/2Z - > Z/2Z by q(x, y) = xy. It is easily verified that q is an R-balanced map and therefore q induces an R-module map q' : Z/2Z $\otimes_{R}^{}$ Z/2Z - > Z/2Z satisfying q(x $\otimes$ y) = xy. Now define an R-map f : Z/2Z - > Z/2Z $\otimes_{R}^{}$ Z/2Z by fx = x $\otimes$ 1. Then q'fx = q'(x $\otimes$ 1) = x, so q'f is the identity. Also fq'(x $\otimes$ y) = f(xy) = xy $\otimes$ 1 = x $\otimes$ 1. Therefore fq' is the identity on a set of generators of Z/2Z $\otimes_{R}^{}$ Z/2Z (in this case these generators x $\otimes$ y are the whole of Z/2Z $\otimes_{R}^{}$ Z/2Z, but that won't always be the case) and we deduce that fq' is also the identity. This proves that Z/2Z $\otimes_{R}^{}$ Z/2Z @ Z/2Z as required.

5. Note that N is finitely generated. This is because M is finitely generated implies M $\oplus$ M is finitely generated, which implies (M $\oplus$ M)/(N $\oplus$ 0) @ N is finitely generated.

   We now apply the structure theorem for finitely generated modules over a PID. We may write

   $$\oplus \oplus \oplus$$ M

   $$\oplus \oplus \oplus$$ N

   
   
   where n, a, b, a_i, b_i are non-negative integers and the q_i are nonassociate prime powers. Since M $\oplus$ M @ N $\oplus$ N, we see that
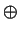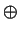   R^2a $$\oplus$$ (R/q₁R)^2a₁ $$\oplus$$ ... $$\oplus$$ (R/q_nR)^2a_n @ R^2b $$\oplus$$ (R/q₁R)^2b₁ $$\oplus$$ ... $$\oplus$$ (R/q_nR)^2b_n.
   By the uniqueness part of the structure theorem, we deduce that 2a = 2b, 2a₁ = 2b₁, ..., 2a_n = 2b_n. Therefore a = b, a₁ = b₁, ..., a_n = b_n and the result follows.

6. (i)

   Note that f has degree 4. Since a_i satisfies f, we have [K(a_i) : K] < 4. Therefore [K(a_i,a_j) : K] < 4*4 = 16, in particular K(a_i,a_j) =/= L as required.

   (ii)

   Assume that f is monic. Then if a $\in$ K is the coefficient of x³ of f, we have a₁ + a₂ + a₃ + a₄ = - a. Since L is the splitting field over K of the polynomial f and we are in characteristic zero, L is a Galois extension of K. Let G denote the Galois group of L over K. Then the subfields of L containing K are in a one-to-one correspondence with the subgroups of G, and G permutes the a_i faithfully. If K(a₁ + 2a₂ + 3a₃) =/= L, then K(a₁ + 2a₂ + 3a₃) corresponds to a nontrivial subgroup of G, in particular a₁ + 2a₂ + 3a₃ is fixed by some nontrivial permutation of the a_i. In other words there is 1 =/= s $\in$ S₄ such that

   a₁ + 2a₂ + 3a₃ = a_s1 + 2a_s2 + 3a_s3.

   By inspection, this will yield a different linear relation from a₁ + a₂ + a₃ + a₄ = - a from above. (Clearly this relation is not 0 = 0. If we subtract the right hand side from the left hand side, then the sum of the coefficients of the a_i is 0.) Therefore we may express the a_i in terms of just two of the a_i; in other words, L = K(a_i,a_j) for some i, j, which contradicts (i). Finally K[a₁ + 2a₂ + 3a₃] = K(a₁ + 2a₂ + 3a₃) because a₁ + 2a₂ + 3a₃ is algebraic over K and the result follows.

7. If the assertion is false, then I(V₁) + I(V₂) is contained in a maximal ideal M of k[x₁,..., x_n]. By Hilbert's Nullstellensatz, M is of the form (x₁ - a₁,..., x_n - a_n), where a_i $\in$ k for all i. Since f (a₁,..., a_n) = 0 for all f $\in$ M, we see that f (a₁,..., a_n) = 0 for all f $\in$ I(V₁) and f $\in$ I(V₂). Therefore (a₁,..., a_n) $\in$ V₁ /\ V₂ which is a contradiction, and the result follows.